Index:
Numbers and Measures in the Earliest Written Records.
Scientific American. February 1984. Volume 250. Number 2. Pages 110-118.
As early as the end of the fourth mlllennium B. C. proto-Sumerian and proto-Elamite scribes had well-developed systems of numbers and measures. They included precursors of our own decimal system.
by Jöran Friberg.
Among the world's earliest written records are inscriptions on clay tablets unearthed in Iraq and Iran, in particular at the sites of two great ancient cities: the early Sumerian city Uruk and the early Elamite city Susa. The inscriptions, mainly accounts and receipts of various kinds, were written toward the end of the fourth millennium B.C. and soon afterward. After more than 100 years of scholarly effort all the systems of numbers and measures in these «proto-literate» texts have now been identified. They turn out to include precursors of the later SumeroBabylonian sexagesimal number system (counting in 10's and 60's) and of our own decimal system (counting only in 10's). In addition they include a previously unrecognized system of capacity measures, used in all accounts dealing with barley, which in this early period was both the basic food grain and the currency.
The reader who would enjoy knowing about the proto-literate systems of numbers and measures will have to join me in a two-directional journey. We shall travel backward in time with respect to the historical record and forward from the past to the present with respect to the scholars who have studied the ancient tablets. The reason we must do so is that the oldest tablets were buried the deepest and were therefore the last to be excavated and made available for study. The oldest tablets were also the most difficult to interpret.
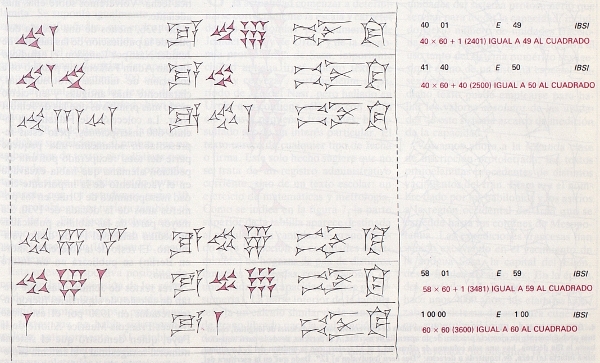
Let us take as our point of departure the Greek coastal island of Cos, some 20 miles northwest of Rhodes. There in about 340 B.C. the founder of a school of astrology, a Babylonian named Berossos, wrote a history of his homeland. In it he told his Greek readers that the numbers sossos (60), neros (600) and saros (3,600) occupied a special place in Babylonian arithmetic and astronomy. Practically nothing more was known about Babylonian numbers and measures for the next 2,200 years. Then in 1855 Sir Henry Rawlinson, one of the pioneers in the decipherment of cuneiform script, published a summary of the cuneiform numbers inscribed on a small clay tablet found at the site of the ancient Mesopotamian city Larsa. Rawlinson realized, among other things, that the last two lines of the tablet stated in effect that «58 1 is the square of 59» and «I is the square of 1». He concluded that the tablet was the final part of an incomplete table of square roots, beginning with the square of 49 (equal to 2,401, or 40 X 60 + 1) and ending with the square of 60 (equal to 3,600, or 60 X 60). His interpretation, of course, was possible only if it was assumed that the numbers 60 and 60 X 60 were both represented by the same symbol, namely the symbol for the number 1.
Rawlinson drew the conclusion that the Babylonians had worked with a sexagesimal number notation of a quasipositional nature, in other words, a number notation in which the symbol for 1 also stood for the powers of 60 and for 10 times the powers of 60. He further concluded that the Babylonians did not have any special sign to represent zero.
Here it is necessary to briefly consider the relative merits of number systems with different bases. Let us begin with the so-called metric system, which is actually a family of interrelated systems of units for several kinds of measures. The metric system owes its current acceptance to its structural simplicity and to the fact that it is constructed to match the base-10, or decimal, system used today for all kinds of routine computations. Since its conception in France in the aftermath of the French Revolution it has gradually spread across the world.
The very length of time it has taken the metric system to gain general acceptance is proof of how difficult it is to suppress other «customary» systems of weights and measures. English examples of such systems include the sequences «mile», «furlong», «chain», «rod», «yard», «foot» and «inch» for measures of length, «barrel», «bushel», «peck», «quart» and «pint» for measures of dry capacity and «ton», «hundred-weight», «pound» and «ounce» for measures of weight. For that matter even the metric system has come to incorporate nondecimal systems: the 12-month year, the 24-hour day, the 60-minute hour and the 60-second minute as units of time, and the 360-degree circle, with its subdivisions the 60-minute degree and the 60-second minute as units of angle. These customary measures can be traced back to classical Greek astronomy and beyond that to the general use of sexagesimal numbers for computation in Babylon and Sumer. Many other customary systems of weights and measures, however, were doomed to be replaced by the metric system because they were insufficiently matched to the widely adopted decimal number system.
Still, the survival of some customary systems has been partially the fault of the decimal number system itself. The decimal system has the weakness that its base of 1O is really too small. This will become more apparent when I give additional examples of computations within the framework of the sexagesimal system with its larger base: 60 = 3 X 4 X 5 (as opposed to 10 = 2 X 5). As will be seen, the base-60 system made it possible for the Sumerians' proto-literate predecessors to construct a family of nicely interrelated measure systems, with sequences of naturally occurring standard units that were easy to deal with in computations.
To find the correct interpretation of the system of cuneiform notations serving to represent sexagesimal (or base-60) numbers was relatively easy. It proved to be much harder to understand how the various systems of measures that appear clearly in many cuneiform inscriptions were constructed. Some decisive clues were offered by certain tablets known to scholars as school texts.
The copying of standard texts was an essential part of the school curriculum in Old Babylonian times (1900 to 1500 B.C.). Many of the texts were lists and tables: lists of geographic names, lists of the names of birds and fishes, lists of words in two languages, grammatical tables for the study of the difficult Sumerian language and so on. Also copied were mathematical tables and lists explaining the structure of the Babylonian systems of measures and their representation in cuneiform script. By doing this kind of copying a student trained himself in cuneiform writing and at the same time accumulated a small personal library of tablets.
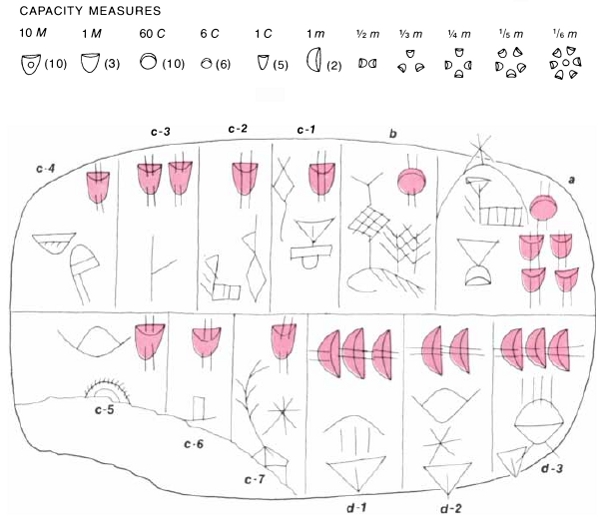
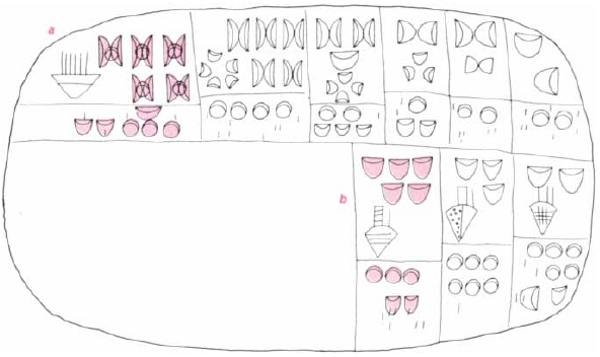
The first example of a table of measures to be described in a scholarly publication was a fragmentary tablet also uncovered at Larsa. The table was discussed by George Smith, a prominent student of cuneiform, in 1872, but its meaning was not fully understood until much later. On the left side of each column of the tablet is a systematically arranged sequence of linear measurements, expressed in standard units. The units are, from the smallest to the largest, the she (a grain), the shu-si (a finger), the kush (a cubit) and so on up to the beru, equal to 30 X 60 X 12 (6 X 602), or 21,600, cubits. On the right side of each column are the same linear measurements expressed as multiples of cubits in sexagesimal notation. For example, the line at the lower right labeled b in the reproduction of the tablet on page 113 reads «Two beru [equals] 12». It should be noted that beru is how the Babylonians pronounced the symbol for the Sumerian word danna (normally written as kas-gid, meaning «long way»). The 12, however, represents not 12 cubits but the much larger sum of 12 X 60|2| cubits. With a cubit being equal to about half a meter in length, the length of the beru was more than 20 kilometers.
When another fragment of the same tablet was identified soon after the first fragment was found, it proved to contain an additional metrological table of the same kind as the first, except that here the right side of each column was concerned with multiples of a nindan (equal to 12 cubits) in sexagesimal notation. Only much later did the study of Babylonian mathematical texts dealing with the computation of volumes make it clear that whereas the cubit was the basic unit for vertical measurements, the nindan was the unit for horizontal measurements. Hence the smallest Sumero-Babylonian unit of area, thie shar, was one square nindan. By the same token the smallest unit of volume, also called a shar, was the space enclosed by a bottom area of one square nindan that had sides one cubit high. This seemingly peculiar choice of units was actually quite practical because it usually excluded the need to count with small fractions of a volume unit.
These two metrological tables are eloquent witness to how well adapted the Sumero-Babylonian system of linear measurements was to the sexagesimal number system. Consider the conversion rules for units of the system of linear measures. Six she is equal to one shu-si, 30 shu-si is equal to one kush, 12 kush is equal to one nindan, 60 nindan is equal to one USH and 30 USH is equal to one kas-gid (or beru). The information contained in this sequence of conversion rules can be condensed as follows: the «conversion factors» for the Babylonian linear system are 6, 30, 12, 60 and 30. Note that each one of these factors is also a numerical factor of the sexagesimal number system. By way of comparison, the Anglo-Saxon sequence from the inch to the mile involves the following conversion factors: 12, 3, 5½, 4, 10 and 8. Whatever the origins of the factors for this customary system, they are clearly in no way adapted to our decimal number system.
Excavations in Mesopotamia have yielded not only mathematically interesting texts such as those from Larsa but also «problem texts» that are even more substantial. As early as 1900 the British Museum reproduced two large Old Babylonian tablets that displayed mixed mathematical problems. Their mathematical terminology was difficult and unusual, and so some 30 years passed before the majority of the problems were interpreted and fully understood. Today a large number of Old Babylonian mathematical problem texts are available for study. To say more about them here would not, however, be within the scope of this exploration of origins. Let me therefore proceed to some other texts published at the beginning of this century.
Between expedition 1889 conducted and 1900 a an major American excavation at Nippur, one of the largest and most important sites in Mesopotamia. By 1906 Herman V. Hilprecht of the University of Pennsylvania had reported some of the expedition's results in a volume that included reproductions of several important Old Babylonian mathematical and metrological texts and a single problem text written in Sumerian. (As recently as a year ago I was able to show that this problem text bears a series of three-dimensional geometric problems solved by reduction to cubic equations and extractions of cube roots).
The Old Babylonian metrological texts Hilprecht published included sexagesimal conversion tables for various kinds of measures and lists of measures from the smallest to the largest. It can be supposed that such lists served to teach both the structure of the Sumero-Babylonian systems of measures and the form of the number signs and other signs belonging to each system.
Hilprecht's publication demonstrated an obvious similarity between on the one hand the Old Babylonian tables and lists and on the other the Sumero-Babylonian tables and lists. Over the years since then quite a number of additional Sumero-Babylonian texts have been unearthed. The oldest of them are a few proto-literate nonmathematical texts that date to the end of the fourth millennium B.C. The oldest mathematical tables are half a millennium younger.
If we now continue our journey backward in time to the beginning of the third millennium B.C. we come to the Sumerian period known as Ur III (2050-1950 B.C.), which immediately preceded the Old Babylonian period. Ur III is known from an enormous number of cuneiform texts. At that time the quasi-positional notation for sexagesimal numbers the Babylonians used most frequently had not yet become common. In the Sumerians' nonpositional notation separate signs stood for 1, 60 and 602, for 10, 10 X 60 and 10 X 602, and so on. Clearly with this kind of nonpositional notation a special sign for zero was not necessary.
Only a few of the many Ur III texts are of any interest because of their mathematical or metrological content. Here I shall mention just a small group of them, concerned with calculations of the amount of seed grain needed to sow fields of a given size when the spacing of the furrows was given as N furrows per nindan. Preserved copies of a kind of Sumerian Farmer's Almanac indicate that grains regularly were dropped in a furrow at intervals of two fingers (shusi), that is, at a rate of 180 grains (equal to a unit of capacity known as a shekel) per nindan. When, as was quite commonly the case, N was equal to 10, that rate could be expressed as precisely one gur (the largest unit of capacity) per bur (the largest unit of area). This example, in which two grains per finger is equal to one shekel per nindan or one gur per bur, shows in what a nice way the various Sumero-Babylonian units of measure were interrelated in spite of their seeming complexity.
Sumerian domination in Mesopotamia during the third millennium was broken by a brief Semitic interlude, beginning with the rule of Sargon of Akkad (2350-2300 B.C.). The existence of mathematical activity in the period of Sargon and his successors, which is hardly to be doubted, is confirmed by a handful of small tablets recording simple but far from trivial geometric exercises. The Sargonic period is exemplified here by a tablet from the city of Umma that gives an account of daily and monthly religious offerings of beer. Its text contains interesting metrological computations. It also clearly shows that number symbols could be written in two ways: either as cuneiform signs, inscribed with the wedge-shaped end of a stylus, or as circular signs made with the blunt end of a stylus.
A few further mathematical texts are known from the Sumerian Presargonic period and from the preceding Early Sumerian Fara period in the middle of the third millennium B.C. The oldest metrological text from the Fara period is of particular interest. It is a table listing the areas of large square fields up to and including a field of (10 X 60 nindan)2. I recently had the opportunity to identify another Fara text as a related geometric exercise. Two other mathematical texts from the Fara period are well known. Both deal with the same division problem involving large sexagesimal numbers. Although such a small sample of texts does not allow any far-reaching conclusions, it seems clear that the early Sumerian teachers, whose work would appear to have been instruction in what might be called applied elementary mathematics, actually excelled in working with fairly abstract problems involving very large or very small numbers or measures, with algorithms for multiplication or division, with area computations based on tables of square areas and so forth. As we shall see, the predilection for working with unrealistically large and small numbers can be traced back as far as the proto-literate period.
How did Sumerian mathematical and metrological systems evolve? Presenting the principal evidence calls for another step backward toward the beginning of the third millennium B.C. In 1928 Stephen H. Langdon of the University of Oxford published a collection of some 200 texts from tablets and tablet fragments that had been excavated a few years earlier at the small site of lemdet Nasr in Iraq. (The lemdet Nasr collection is now divided between the Ashmolean Museum in Oxford and the Iraqi Museum in Baghdad). The new inscriptions were written in an archaic pictographic script that was recognizably a precursor of Sumerian cuneiform. Many of the signs in the archaic texts, however, were no longer in use by the time the cuneiform texts of the Early Sumerian Fara period were written.
For that reason even today the correct reading of many signs in the «proto-Sumerian» inscriptions from lemdet Nasr is still not known and the texts remain more or less unintelligible. It is not even completely clear that the language of the lemdet Nasr tablets is Sumerian, which is why I refer to the inscriptions as proto-Sumerian. Nevertheless, the numbers that appear in these texts, as a rule made with the round end of styluses, are always easy to identify. It was also immediately recognized that the number notations on the lemdet Nasr tablets were closely related to those on even more enigmatic tablets from Iran. These tablets are for the same reason called proto-Elamite, and they are of about the same age. We shall be returning to them.
In 1936, less than a decade after Langdon's publication of the lemdet Nasr tablets, the German scholar Adam Falkenstein published another collection of proto-Sumerian tablets, clearly older and somewhat more primitive than those from lemdet Nasr. Falkenstein's collection included 600 inscriptions, but this was only a small part of the total excavated by a German expedition that had worked at the site of the important Mesopotamian city of Uruk in the early 1930's. The majority of the published tablets came from Level IV of the site. The balance of the collection (now in Berlin) is scheduled for publication in the near future.
Three texts from Jemdet Nasr dealing with area computations were interpreted in 1930 by the French scholar François-Maurice Allotte de la Fuye, who was able to prove that the sexagesimal number system and the Sumerian system of area measures were also used in these proto-Sumerian inscriptions. With this exception, however, proto-Sumerian notations for numbers and measures continued to be little understood. It was generally assumed, however, that along with the Sumerian sexagesimal system the early scribes resorted to a decimal (or «centesimal») number system, possibly borrowed from the proto-Elamite. It was also assumed on loose grounds that in the proto-Sumerian grain texts (characterized by the sign she, meaning grain) the main capacity unit was a gur consisting of 300 sila (or 30 ban), just as in the grain texts of the later period Ur III.
In 1978 I was able to demonstrate the falsity of these assumptions by proving that in both kinds of proto-literate grain texts, proto-Sumerian and proto-Elamite, the number sign invariably read by scholars as 10 is just as likely to have the value 6. As a matter of fact, even in a single text the same number sign can be read either as 10 or as 6, depending on the context.
This new insight at once made it possible to understand the computations in hundreds of proto-Sumerian and proto-Elamite capacity-measure texts that before had been impossible to analyze. By drawing parallels between certain classes of proto-literate texts and their Sumerian counterparts I was further able to determine, at least approximately, the absolute size of the main proto-literate unit of capacity, which turned out to be about one Sumerian ban (equivalent to 10 liters) rather than the assumed 30 ban.
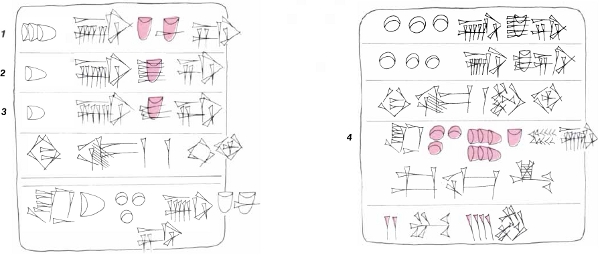
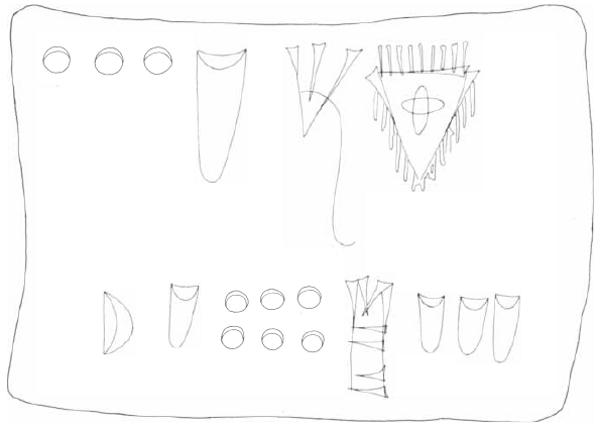
As a result of these advances it is now possible to begin to correctly assess the scale and character of the proto-Sumerian economies at Jemdet Nasr and Uruk and of the proto-Elamite economy at the important ancient Iranian city of Susa. Thus, for instance, a certain «bread and beer» text belonging to the Jemdet Nasr period but found at Uruk that contains computations with large numbers and small fractions has proved to be of particular interest. The text lacks any kind of date or signature. This fact alone suggests that it is no ordinary administrative record but a school text: an exercise in mathematics and metrology. As is indicated by the bottom illustration on this page, the upper part of the tablet is devoted to the computation of the amount of grain expended in baking given numbers of loaves of bread of various sizes (indicated by fractions of a minor proto-Sumerian capacity unit). The lower part of the tablet does a similar calculation for the quantity of grain expended in brewing two jars of strong beer, three jars of medium-strength beer and five jars of weak beer. The different strengths of the three batches of beer are evidenced by the different quantities of grain per jar in the three instances.
This particular example of a proto-Sumerian text of the lemdet Nasr type is important for several reasons. First, it establishes beyond doubt the relative values of quite a few units of the proto-Sumerian system that served for measuring capacity, including a number of fractional units. Second, it demonstrates the use of both the sexagesimal number system and a special «bi-sexagesimal» system. Equally important, the text can be used to find the absolute values of the units of this archaic system of measuring capacity.
Let us turn now to the second kind of proto-literate inscription, the proto-Elamite texts from various sites in Iran. Elam was the name given by the Babylonians and the Assyrians to the western region of Iran extending to the borders of Mesopotamia. French expeditions have been excavating at the site of ancient Susa, a capital city of Elam, since late in the 19th century. At the time of the Old Babylonian period, almost 4,000 years ago, the Elamites were using the Sumero-Babylonian cuneiform system of writing. Excavations at Susa and in other parts of Iran have revealed, however, the existence of an earlier Iranian civilization that wrote a noncuneiform script and flourished for a brief period a millennium before the time of the Old Babylonians and the Elamites. This proto-Elamite civilization was vigorous enough to extend its influence beyond Elam itself northward and eastward to distant corners of the Iranian plateau.
Hundreds of archaic clay tablets from Susa (they constitute the greater part of all known proto-Elamite texts) were published by the French scholar Vincent Scheil between 1900 and 1935. Scheil was also the first scholar to note one parallel between the Sumerian and the proto-Elamite scripts: the method of producing numerical notations. Otherwise, however, the proto-Elamite script shows no relation to any other known script. Its repertory of signs, representing the words or syllables of an unknown language, is also unhelpfully abstract and nonpictographic, a circumstance that would seem to make interpretation of the non-numerical parts of the proto-Elamite texts virtually impossible.
Scheil's first attempts to understand the nature of the number systems in his proto-Elamite texts were unfortunately not very successful. He failed to recognize that one sign a small circular impression-need not always have the numerical value of 10. He was thus for a time led to the conclusion that the proto-Elamites, like the ancient Egyptians, worked with a decimal number system in all their accounts and computations. Later, however, he found that some proto-Elamite texts actually feature a special sign for 60. Scheil also identified a series of fractional notations and went on to correctly identify the proto-Elamite ideograph for «grain» or perhaps «grain measure».
A proto-Elamite mathematical-metrological exercise text, published by Scheil in 1935, deals with the addition of a long series of numbers, made up of many digits and representing measures of capacity. This particular text yields excellent confirmation of my own analysis of the structure of the proto-Elamite units for measuring capacity. The system was clearly constructed to match the sexagesimal (or bi-sexagesimal) number system; it has the following sequence of conversion factors from one unit to the next: 6, 10, 3, 10, 6, 5, 2, 3, 2, 2. The corresponding series of conversion factors for the units of the proto-Sumerian system, omitting the first factor and the last four, is 10, 3, 10, 6, 5. Hence the two systems differ from each other only in their respective ways of representing fractions of the small capacity unit (a measure that corresponds to the liter in the metric system).
I wrote an account of this analysis of the two systems' units of capacity measure in a 1978 research report. The same report included another surprising discovery, namely that the proto-Elamites (but not the proto-Sumerians) used the sexagesimal number system only when they were counting people or inanimate objects such as loaves of bread or clay vessels. When they were counting animals, they worked with a decimal number system!
The use of the decimal system for counting animals is confirmed by a famous proto-Elamite text published by Scheil in 1923. The text is uncharacteristic: it includes unmistakable pictographs rather than the customary obscure ideographs. The heads of horselike animals are displayed in four different categories, probably divided according to sex and age. There can be no doubt, however, that the «horses» were counted with decimal numbers. To add to the general confusion resulting from the proto-Elamite use of two different number systems for counting, several of the proto-Elamite signs for numbers (not only the infamous small circular impression) have different values depending on the context. For example, the sign for 1,000 is the same as the one for 2 X 60.
To summarize, as a result of my identification of the systems used in the proto-literate texts for numbers and measures, it is now possible for the first time to divide the archaic proto-Sumerian and proto-Elamite numerical texts so that they fall into a comparatively small number of categories according to their content. The content of a text is indicated in particular by the kind of numbers and measures used in it. For example, among the proto-Elamite texts the main categories are enumerations of people (sexagesimal counting numbers), computations of grain expended on rations for people (capacity numbers and sexagesimal numbers) or for animals (capacity numbers and decimal numbers), bread-and-beer texts (capacity numbers and sexagesimal numbers), accounts of groups of animals (decimal numbers), accounts of loaves of bread or clay vessels (sexagesimal numbers) and accounts of large quantities of grain delivered to or dispatched from storehouses, in one case during a five-day «week» (capacity numbers). Additional categories are to be found among the proto-Sumerian texts: area computations (area numbers and sexagesimal numbers) and in a single instance a seed-grain text (area numbers and capacity numbers).
Much work remains to be done before the existing collections of proto-literatetexts, published or still unpublished, can be persuaded to give up all the information they contain. Nevertheless, so much progress has been made, particularly in the past decade, that it is now at last feasible to sketch a plausible outline of the development of numeration and metrology in southwest Asia from prehistoric to Late Babylonian times.
In particular it is now known, from the work of Denise Schmandt-Besserat of the University of Texas at Austin, that a variety of clay «tokens» served in the region continually from the ninth millennium B.C. to the ofend the fourth millennium to designate numbers, measures and perhaps categories of objects. It is also known that the use of loose tokens was complemented late in the fourth millennium by the device of enclosing selected symboIs in protective envelopes of clay, many of them bearing surface impressions resembling the enclosed tokens. The envelopes in turn seem to have inspired the invention of «impressed tablets». (The term refers to clay tablets bearing number notations and usually cylinder-seal impressions but lacking any abstract or pictographic word signs).
The similarity of the form of the number signs on the impressed tablets and the shape of the earlier clay tokens show a continuity of representations of numbers and measures from the time of the rise of city-states in southwest Asia backward in time to the early Neolithic, as long as 10,000 years ago. Moreover, the fact that notations for units belonging to various proto-literate measure systems also clearly appear on some impressed tablets shows a continuity in representations of numbers and measures forward in time from late in the fourth millennium B.C. all the way to the Old Babylonian period, which was some 2,000 years later. And, as we have seen, our own decimal system is a reflection of the same continuity down to the present day.
The illustrations.